Wednesday, April 30, 2003

Examples of Linear Models

• simple linear regression
• one way analysis of variance
• analysis of covariance

Reading

Chapter 11 and class notes.

Homework

Problem: Let 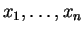$x_1,\dots,x_n$ be constants, and suppose

$$Y_i = \beta_1 + \beta_2 x_i + \varepsilon_i$$

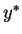Let $y^*$ be a constant and let let 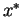$x^*$ satisfy

$$y^* = \beta_0 + \beta_1 x^*$$

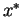that is, $x^*$ is the value of 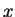$x$ at which the mean response is $y^*$.

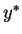a.

Find the maximum likelihood estimator 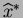$\widehat{x}^*$ of $x^*$.

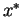b.

Use the delta method to find the approximate sampling distribution of $\widehat{x}^*$.

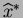Due Friday, May 2, 2003.